The critical value is a crucial topic in statistics. No matter what statistical study you’re doing or conducting, critical values will always come in handy, and you’re going to need a thorough concept of critical values. But don’t worry, they’re not as tricky as they might seem
initially.
So today, we will be going over the basics of critical value with you so that by the end of this article, you can easily calculate the critical value for anything on your own.
Below is the agenda for our today’s discussion:
- What Is A Critical Value
- Steps For Calculating Critical Value
- The Importance of Critical Value
- Several Types of Critical Value Systems
So, let’s get right into it without wasting any time!
What Is a Critical Value?
Whilst performing a statistical test, one must calculate the confidence interval followed by the upper and lower bounds. These upper and lower bounds are called the critical values. The critical values tell you how far you have to move from the means to produce a notable change in the data. In other words, the critical values can also be considered as the “margins of errors” of a dataset.
The Three Different Types of Critical Value Systems:
In order to obtain reliable and valid results from your statistical tests, there are three different critical value testing systems available that will tell you if the results of your calculations are valid:
Chi-squared:
C = Degree of freedom
O = Observed values
E = Expected values
How to Calculate Critical Value of Chi-squared?

Chi-squared tells you about the relationship between two different categorical variables. One of these variables is a numerical (countable) one, whilst the other is a non-numerical (categorial) one. The result from the chi statistic tells you the difference between the observed and the expected counts through a single number.
This method is not the most favorable method because you have to sum up the calculations for every possible value of O and E, and this makes this method tedious. Now a few variations of this method are available. These variations depend upon the method of your collection.
T-scores:
X (bar) = Mean of the sample data
μ0 = Mean of the population
S = Sample of the standard deviation
N = Size of the sample
How to Calculate Critical Value of t?
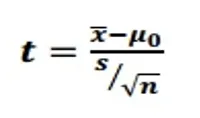
T-scores are a method of developing your own standard of checking similar samples. The larger the value of the t-score, the more significant the difference between the groups you are testing.
For example: In a relative grading system, the papers are graded according to the average score attained, the maximum, and the minimum scores, unlike the absolute grading system where there is a pe-set grading criterion.
Z-scores:
X = Values from the sample
μ = Mean value of the sample
σ = Standard deviation of the sample
How to Calculate Critical Value of Z?
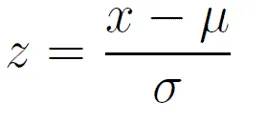
The z-score, which is also known as the “standard score“, gives you an idea of the distance of a point from the mean value. In complex terms, it tells you the counts of standard deviations a score above or below the mean value.
For example, you know someone’s IQ level and want to find out how different that is from an average person’s IQ level. There are many levels of an average person’s intelligence, and the z-score will make it easier for you to determine how far someone’s IQ level is from an average person’s IQ level.
How To Calculate Critical Value in Statistics?
In order to check other characteristics of a dataset or test its validity, it is essential to know what the margins of errors for that dataset are.
For Example: If you want to determine how a certain medicine affects an average person, you need to test a group of people and calculate the bounds for the errors in your calculations to ensure that there are no discrepancies in your research.
The critical values for any dataset can be calculated through the formula:
Critical probability (p) = 1 – (Alpha / 2)
Alpha = 1 – (the confidence level / 100)
The answer to this formula can be either a z-score using the cumulative probability or a critical t-statistic.
1. Computing the alpha value
Before calculating the critical probability, you must first calculate the value for alpha through the formula given above. The confidence level, in this case, is the values above or below a statistical parameter for which the calculations will remain true. Typically, this value is represented in percent form.
For Example: The confidence level of a dataset if 88% then the value of alpha will become [alpha = 1 – (88 / 100) = 0.12]
2. Calculating the critical probability
Now that you have the value for alpha, you can calculate the critical probability by putting the value of alpha in the formula for the critical probability. Afterward, you can express it as a t-statistic or a z-score, depending on the size of the samples.
In this case: critical probability (p) = 1- (0.12/2) = 0.94 or 94%
The Importance of Critical Value
Critical values play an incredibly significant role in evaluating the accuracy of your research or the validity and checking the range for errors. These values tell you about the different characteristics of your dataset and how it behaves at certain points.
If you happen to work with a small dataset, it’s essential you express the critical values as a t-statistic because the standard deviation is unknown. Likewise, when you are working with a larger dataset (with sets of more than 40), it is better if you express your data in the form of cumulative probability or the Z-score for a better evaluation.
Learn More about Statistics
-
Bootstrapping vs. Boosting
Over the past decade, the field of machine learning has witnessed remarkable advancements in predictive techniques and ensemble learning methods. Ensemble techniques are very popular in machine learning as they
-
What Are the Three Criteria for Causality?
Understanding the process, how things work, how one event leads to the other, or the famous concept of the butterfly effect, all these ideas relate to causality in one way
-
What are the Data Collection Tools and How to Use Them?
Ever wondered how researchers are able to collect enormous amounts of data and use it effectively while ensuring accuracy and reliability? With the explosion of AI research over the past
Conclusion
Critical values are of great worth in the world of statistics since they allow you to find out the boundaries of your datasets, and along with that, they tell you how much you can rely on the results from the calculations you just performed on that dataset.
We hope that by now, you have become familiar with the steps that should be taken to calculate the critical value of any data set given to you and that you have also understood the importance of calculating critical values. For more information on this topic, please click here.

